AP® Statistics: 6 Math Functions You Must Know for the TI-Nspire™ CX Graphing Calculator
With schools opening up in spring of 2021, students and teachers are getting prepared for another unique year on AP® Statistics exam administration. This year, as in every year, several features of the TI-Nspire™ CX graphing calculator will be useful for you. In this post, I have compiled a list of six of the most important statistics features to know for the TI-Nspire™ CX graphing calculator.
1. Regression Analysis
It is unlikely that you will be asked to draw a scatterplot on the exam; however, being familiar with creating and analyzing a scatterplot of bivariate data could prove crucial. Your data should be stored in a Lists & Spreadsheet page in named lists. One of the key advantages of the TI-Nspire™ CX graphing calculator is that you can use representative names for the lists of data. A Data & Statistics page allows for quick, interactive graphing of the data. From here, a quick look at the residual plot helps to confirm whether the computed regression model fits the data well. Refer to the video below for how to do this on your calculator.
2. Normal Probabilities
The computation of probabilities for random variables that are normally distributed is a core concept in the course. You can expect a question involving a normal probability computation on the AP® Statistics exam. Using the Probability menu on a calculator page and choosing statistics, the normal cdf command takes inputs of lower and upper boundary, as well as the mean and standard deviation. This is helpful because it means you do not need to standardize (turn to z-scores) the values before using the command. Since you are allowed boundary values, you also don’t have to apply the complement rule. However, do make note that the default values for the lower boundary, mean and standard deviation, do assume you have standardized the values. To find the probability that X takes a value between 65 and 86, if X is normally distributed with mean 70 and standard deviation 15, follow the steps in the video below.
3. Binomial Probabilities
You know the verbal cues to recognize when a question is about a binomial context, but which probability distributions menu item do you use for a given situation? If the question is asking for the probability of a single outcome (in other words, what’s the probability of exactly five heads in 10 coin flips [Hint: It’s not 1/2!]), then you should use Binomial Pdf with number of trials, n: 10, probability of success, p: 0.5, and X Value: 5. If the question is asking about the probability of more than five heads in 10 coin flips, then you need Binomial Cdf. Keep in mind that Binomial Cdf is cumulative from 0 to k successes, so pay close attention to whether to subtract the endpoint, or not, when using the complement rule. You can also use the lower boundary if you need to find the cumulative probability of success between two values. Always remember that for the free-response section, you must show the correct work and justification for the computation. The exam readers will not accept “calculator speak” as justification; in other words, “1 - binomcdf(10,.5,5)” is not considered sufficient communication of work. The video below shows an example of this kind of computation.
4. Random Variables
In some cases, you are asked to calculate the mean or standard deviation of a random variable given in a table. You can use a little-known feature of statistics calculations. With the random variable in one list, and the frequencies in another list, you can calculate the mean of the random variable by using the command mean (RVname,Freqname). The video below shows an example of this computation.
5. Checking Conditions
Rarely on the exam will you be asked directly to make a graph of univariate data. But, when checking the conditions for an inferential procedure involving means, knowing quickly how to generate a graph of data, and determine its relative shape, is important. Use a boxplot or a histogram to assess the shape of a sample distribution for strong deviations from approximately normal. More steps are included in the video below.
6. Inferential Procedures
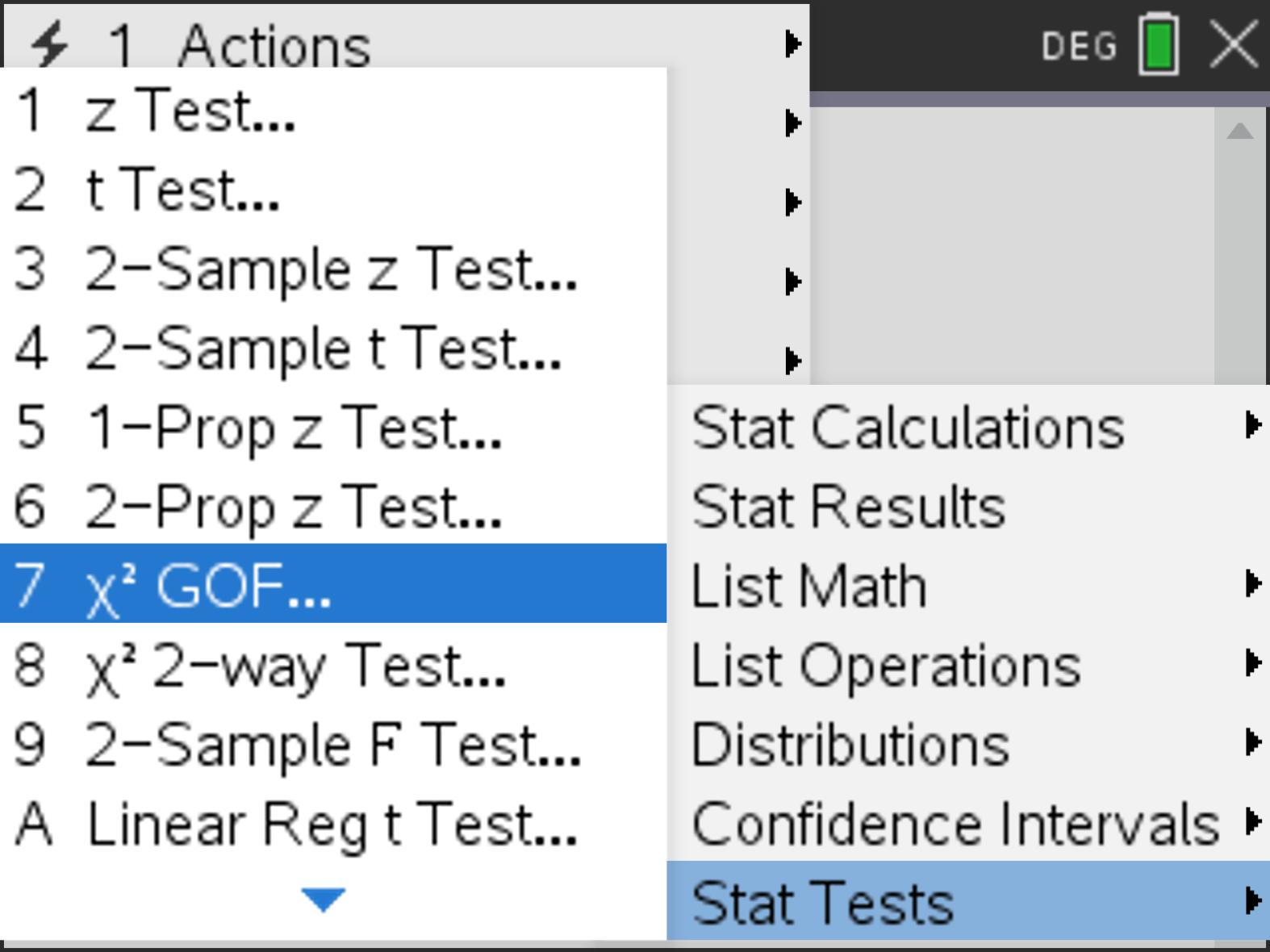
As sure as the sun will rise tomorrow, you will be asked to carry out an inference procedure on the free-response portion of the exam. You may be given sample statistics to work with or perhaps a small data set to input. Either way, you must be sure to know which type of inferential procedure to do. Key words like “provide evidence” or “significant” are indications that you are being asked to test a pair of hypotheses. “Estimate” or “confidence” gives indication that an interval is appropriate to answer the question. Be sure familiarize yourself with each type of inferential procedure, including its outputs. Recall that the t-procedures are required when dealing with questions about a population mean or difference in two population means. Inference procedures for proportions are indicated by “Prop” in the title of the command on the calculator. A Chi-squared test for homogeneity has the same mathematics as a Chi-squared test for independence, so there is only one Chi-squared test option for these tests, Chi-squared 2-way Test. The Stat Tests menu can be found by pressing menu, then choosing Statistics, and navigating to the option Stat Tests.
AP® is a trademark registered by the College Board, which is not affiliated with, and does not endorse, TI products. Policies subject to change. Visit www.collegeboard.org.
About the author: Curtis Brown currently works as the Math Segment Manager at Texas Instruments (TI). He taught mathematics and AP® Statistics for several years. Since starting at TI in 2015, he has led many content development projects including the Math in Motion series. He has always found joy in exploring the patterns and logic of mathematics. In his spare time, he enjoys kayaking and fishing with his sons and spending time with his family. Follow him on Twitter and Instagram @cbmathguy.
Tagcloud
Archive
- 2025
- 2024
-
2023
- January (3)
- February (3)
- March (5)
- April (3)
- May (3)
- June (3)
- July (2)
-
August (6)
- 5 Ways to Spruce Up Your Classroom for Back to School
- Day of the Dog: Which Dog Is Roundest?
- Women Who Code: A TI Intern’s Fascinating STEM Journey
- 6 Sensational TI Resources to Jump-Start Your School Year
- 3 Back-to-School Math Activities to Reenergize Your Students
- A New School Year — A New You(Tube)!
- September (2)
- October (3)
- November (1)
- 2022
-
2021
- January (2)
- February (3)
- March (5)
-
April (7)
- Top Tips for Tackling the SAT® with the TI-84 Plus CE
- Monday Night Calculus With Steve Kokoska and Tom Dick
- Which TI Calculator for the SAT® and Why?
- Top Tips From a Math Teacher for Taking the Online AP® Exam
- Celebrate National Robotics Week With Supervised Teardowns
- How To Use the TI-84 Plus Family of Graphing Calculators To Succeed on the ACT®
- AP® Statistics: 6 Math Functions You Must Know for the TI-84 Plus
- May (1)
- June (3)
- July (2)
- August (5)
- September (2)
-
October (4)
- Transformation Graphing — the Families of Functions Modular Video Series to the Rescue!
- Top 3 Halloween-Themed Classroom Activities
- In Honor of National Chemistry Week, 5 “Organic” Ways to Incorporate TI Technology Into Chemistry Class
- 5 Spook-tacular Ways to Bring the Halloween “Spirits” Into Your Classroom
- November (4)
- December (1)
-
2020
- January (2)
- February (1)
- March (3)
- April (1)
- May (2)
- July (1)
- August (2)
- September (3)
-
October (7)
- Tips for Teachers in the time of COVID-19
- Top 10 Features of TI-84 Plus for Taking the ACT®
- TI Codes Contest Winners Revealed
- Best of Chemistry Activities for the Fall Semester
- Best of Biology Activities for the Fall Semester
- Best of Physics Activities for the Fall Semester
- Best of Middle Grades Science Activities
- November (1)
- December (2)
- 2019
-
2018
- January (1)
- February (5)
- March (4)
- April (5)
- May (4)
- June (4)
- July (4)
- August (4)
- September (5)
-
October (9)
- Art in Chemistry
- Which Texas Instruments (TI) Calculator for the ACT® and Why?
- Meet TI Teacher of the Month: Jessica Kohout
- Innovation in Biology
- Learning With Your Students
- A first-of-its-kind STEM strategy charts path to help educators
- #NCTMregionals Hartford 2018 Recap
- The Math Behind “Going Viral”
- Real-World Applications of Chemistry
-
November (8)
- Testing Tips: Using Calculators on Class Assessments
- Girls in STEM: A Personal Perspective
- 5 Teachers You Should Be Following on Instagram Right Now
- Meet TI Teacher of the Month: Katie England
- End-of-Marking Period Feedback Is a Two-Way Street
- #NCTMregionals Kansas City 2018 Recap
- Slope: It Shouldn’t Just Be a Formula
- Hit a high note exploring the math behind music
- December (5)
- 2017
- 2016
- 2015
