Investigate interior angles of polygons and the sum of their measures.

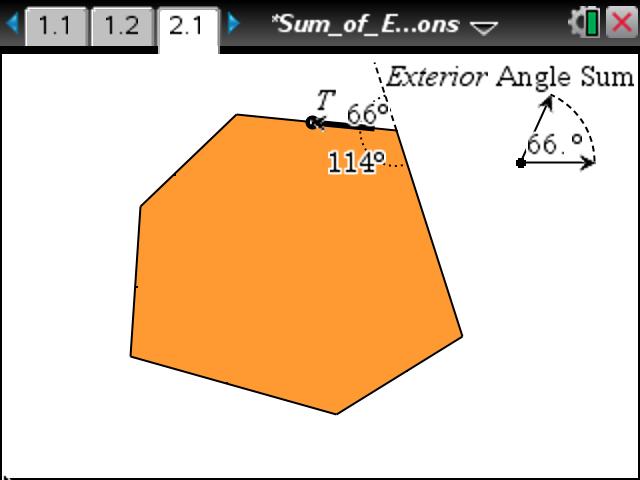
• Determine that the interior angle of a polygon and an exterior angle of a polygon form a linear pair (i.e., the 2 angles are supplementary)
• Determine that if one exterior angle is drawn at each vertex of a convex polygon, then the sum of the measures of those exterior angles is 360°
• Determine a formula for the measure of one exterior angle of a regular polygon and use this to discover an alternative form for the formula that is typically used to calculate the measure of the interior angle of a regular polygon

• exterior angle of a polygon
• regular polygon
• irregular polygon
• interior angle of a polygon

This lesson involves moving an arrow along the side of regular and irregular polygons to form an exterior angle with the adjacent side of the polygon. As a result, students will:

• Discover that an interior and exterior angle of a polygon form a linear pair.
• Determine that the sum of the measures of the exterior angles of any convex polygon is 360°.