Energy in Simple Harmonic Motion
Energy in Simple Harmonic Motion
In this lesson, students will simulate a mass attached to a spring as it oscillates in simple harmonic motion.
- Students will analyze energy transformation for a mass attached to a spring as it oscillates in Simple Harmonic Motion.
- Students will explore the Conservation of Energy principle.
- conservation of energy
- gravitational potential energy
- kinetic energy
- spring potential energy
- total mechanical energy
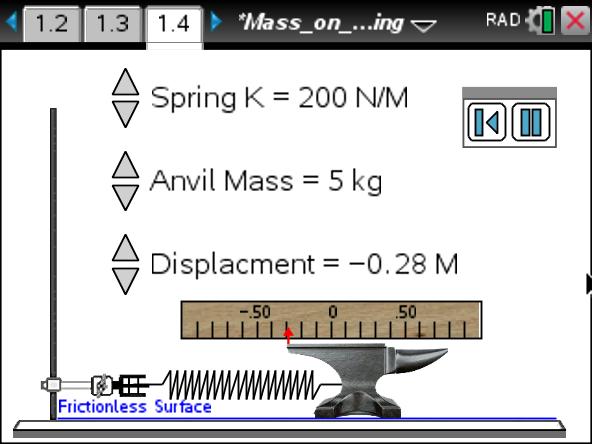
This lesson simulates a mass attached to a spring as it oscillates in simple harmonic motion. In the first part of the lesson the mass oscillates across a frictionless, horizontal surface. In the second part, the mass is suspended from the spring and oscillates vertically. Bar graphs represent the potential and kinetic energies of the system.
As a result, students will:
- Observe how energy transforms between potential energy and kinetic energy as the mass moves through its cycle.
- Describe factors that affect the total energy of the system and the maximum speed of the mass.
- Observe that the total energy remains constant throughout the motion.
Vernier EasyData,Vernier EasyLink and Vernier EasyTemp are registered trademarks of Vernier Science Education.

