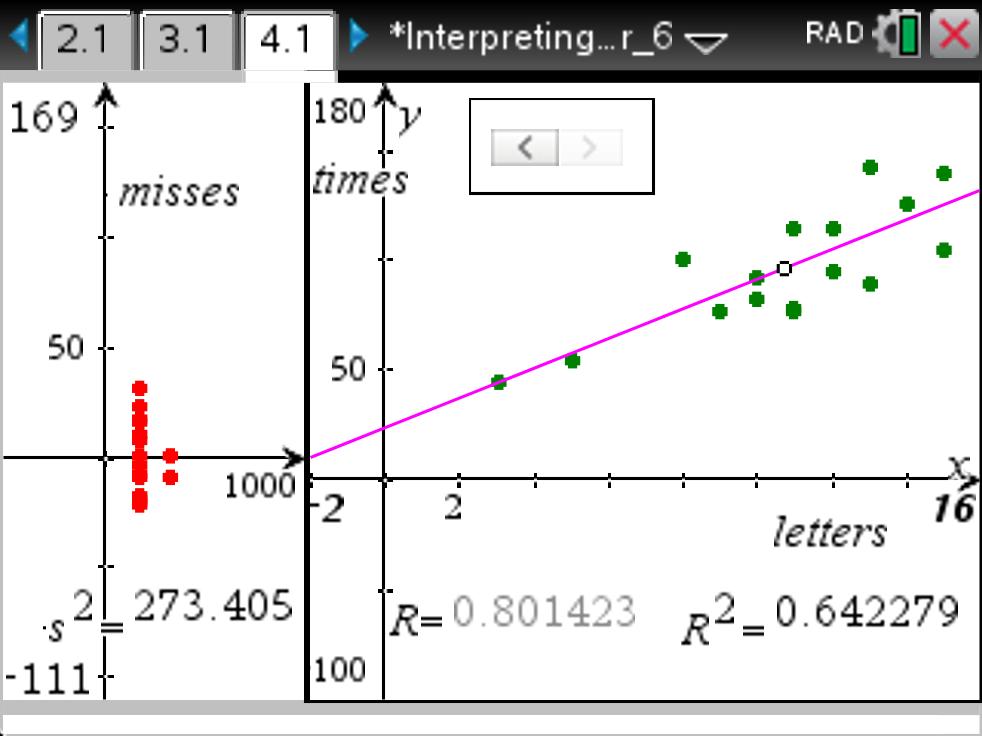
This lesson involves predicting values of a particular variable.

• Students will predict a response variable using the mean of that response variable and recognize why the mean of the response variable minimizes the typical "error" in the prediction if no other information is available.
• Students will recognize that R² measures the relative improvement in precision in predicting a response variable using an additional (explanatory) variable via the least-squares regression line (over the precision obtained using only the mean of the response variable).

• centroid
• coefficient of determination
• dot plot
• linear correlation coefficient
• linear regression
• mean
• residual
• scatterplot
• variance

This lesson involves predicting values of a particular variable.

As a result, students will:

• Examine both univariate and bivariate displays involving a particular variable in order to visualize the errors made by different methods of predicting that variable.
• Consider various measures that might be used to quantify the precision of a particular predictor of values of a given variable.
• Use percent decrease in the adopted measure of precision as a measure of improvement from one method of predicting to another.
• Make and verify a conjecture describing the amount of improvement in the precision of predicting a response variable when comparing the least-squares prediction line to predictions using only the mean of the response variable.