Understanding
A model that "fits" the data well minimizes the sum of the absolute deviations from line.
What to look for
To analyze how well a linear equation "fits" the data, construct a scatter plot of the residuals for any patterns or predictability. Models that result in a pattern in the residual plot do not model the data well.Sample Assessment
The scatter plot below shows the relationship between height, in centimeters, and the arm span, in centimeters, of 15 students in a class.
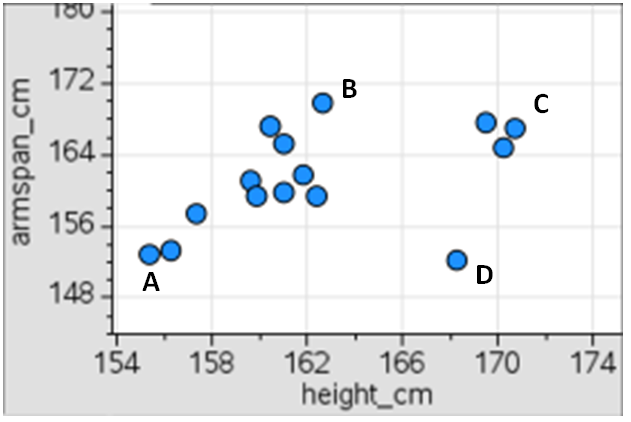
Based on the scatter plot, determine which ordered pair would be the farthest from the best fit line.
a) A
b) B
c) C
d) D
Answer: D




