Expressions and Equations / Prop. Relationships to Linear Equations

Understanding

This lesson helps students to use the point slope form of a line to move from graphs of proportional relationships to graph of any line.

What to look for

Student discussion may provide an opportunity to discuss why the denominator of a fraction cannot be zero.

Sample Assessment

Consider the graph of a line.

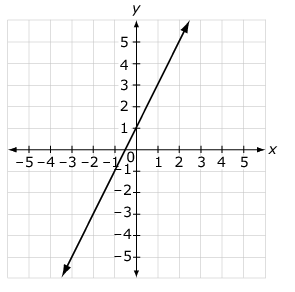
Which equation has a slope greater than the slope for the line shown?

a. \(y = 3x-1\)      b. \(y = \frac{x}{2}+4\)

c. \(y = 2x+2\)      d. \(y = \frac{x}{3}-3\)

Answer: a

The Big Idea

Any sequence of multiplications may be calculated in any order and the numbers may be grouped together any way.

What are students doing?

They explore different sets of factors that produce the same result and consider whether an exponent can “distribute” over any operation.

What is teacher doing?

Encourage students to explore equivalent ways of writing numerical expressions involving exponents.