1. Use the fnInt command to graph one member of the family of curves represented by 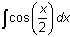and interpret the result.
2. Use analytical methods to find the general solution to the differential equation y' = e^3x.
3. Use EULERG to graph the solution to the initial value problem y' = y(10 - y) and y(0) = 1. Define a [0, 1, 1] x [0, 15, 5] window and use a step size of 0.01.
4. If y' = ln(x) and y(1) = 2, use EULERT to estimate y(3). Use a step size of 0.25.
5. Graph the slope field for the differential equation 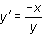using a [-3, 3, 1] x [-2, 2, 1] window.

Click here to check your answers.