One antiderivative appears to be
![]() ,
as confirmed by the graph of Y2 = X4 / 4 coinciding with the graph of
,
as confirmed by the graph of Y2 = X4 / 4 coinciding with the graph of
![]() .
.
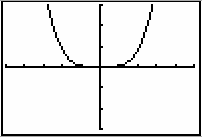 |
|
Y1 = fnInt(T^3,T,0,X) Y2 = X^4/4 |
![]()
For x = 1 and y = 1,
![]()
Therefore, ![]() .
.
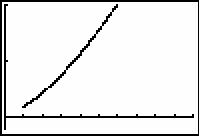
The graph shown is that of
![]() ,
the solution of
,
the solution of
![]() with y(1) = 1 which was found earlier.
with y(1) = 1 which was found earlier.
You need 20 points to reach y(1)
![]() 1.95.
1.95.
y(1.2)
![]() 1.2073. The actual value rounds to 1.2097.
1.2073. The actual value rounds to 1.2097.
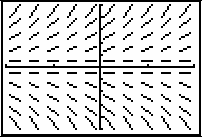
In this slope field the slopes of segments in a given row are equal. In the previous slope field the slopes of segments in a given column were equal. This difference is due to the fact that the differential equation in this problem is a function of y, therefore the slopes depend on y, not x.
Y1 = fnInt(cos(T/2),T,0,X)
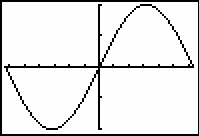
[-2
Any integral of the form
![]() is a member of the family of curves represented by
is a member of the family of curves represented by
![]() .
.
The graph shown above is the graph for a = 0. Other values of a will produce vertical shifts of this graph.
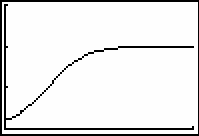
[0, 1, 1] x [0, 15, 5]
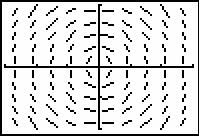
[-3, 3, 1] x [-2, 2, 1]
©Copyright 2007 All rights reserved. | Trademarks | Privacy Policy | Link Policy