A related-rate problem that models two ships as they move away from each other is discussed in this lesson.

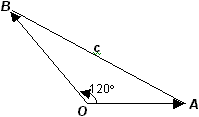
Two ships start at a point O and move away from that point along routes that make a 120° angle. Ship A moves at 14

A knot is a unit used to measure the speed of a ship. One knot represents one nautical mile (6,076.1 feet) an hour.

knots and Ship B moves at 21 knots. In the diagram below A represents the position of Ship A, B represents the position of Ship B, and c represents the distance between the ships.

Modeling the Positions of the Ships

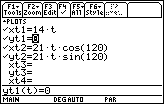
Suppose that Ship A is moving along the positive x-axis at 14 knots. Enter the parametric equations for Ship A's position at time t hours.

• Set xt1 = 14t
• Set yt1 = 0

Parametric equations can be used to model the position of an object at time t that is moving at speed v along a line that forms an angle with the positive x-axis. Such equations have the form

x = vt cos
y = vt sin

Enter the equations for Ship B's position, which is moving at 21 knots along a line that forms a 120° angle with the path of Ship A.

• Set Angle mode to DEGREE
• Set xt2 = 21t*cos(120)
• Set yt2 = 21t*sin(120)

Animating the Motion of the Ships

• Change the Graph Style of both sets of equations to "Path"

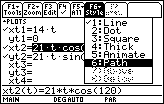
Make sure the Graph Order in the Graph Formats dialog box is still Simultaneous.

• Enter the Viewing Window values shown below:

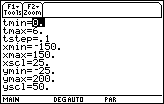
• Graph the equations

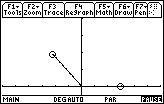
Finding the Distance between the Ships

The distance c(t) between the ships can be found by using the distance formula:

Use the restriction t 0 because the problem starts at t = 0.

• Define
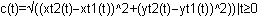
• Enter c(t) on the Edit Line and press

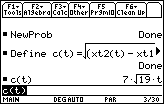
Thus, .

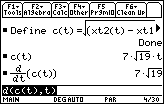
Finding the Speed at which the Ships are Moving Apart

The speed at which the ships are moving apart can be found by finding the derivative of c(t) with respect to t, or just observing that it is knots from the formula for c(t).

14.3.1 Approximately how far apart are the ships after two hours? Click here for the answer.

Modifying the Problem

Assume that both ships travel in the same direction and at the same speed as before, but Ship A begins its journey 5 nautical miles from point O and Ship B begins 3 nautical miles from point O.

• Set    xt1 with 14t+5
           xt2 with (21t+3)*cos(120)
           yt2 with (21t+3)*sin(120)

14.3.2 Find the derivative of c(t). Click here for the answer.