Curves described using polar coordinates can be very interesting and the equations are often much simpler in polar form than they are in rectangular form. This lesson explores graphing polar equations.

When graphing polar functions on the TI-83,

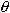1. is the independent variable and r is the dependent variable.
2. Angles are positive if measured in the counterclockwise direction from the positive x-axis.

Viewed as function variables, the order of the polar coordinates (r, ) is reversed compared to rectangular coordinates (x, y) where the independent variable is first.

Graphing in Polar Mode

• Change to Polar Graphing mode.

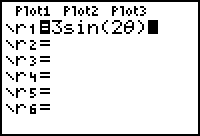
Graph the polar functions r = 3 sin(2 ).

• Open the Y= editor by pressing . The function editor in Polar Graphing mode is still called the Y= editor, even though the functions are denoted by r1, r2, and so forth.
• Set r1 = 3sin(2 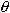). Press for .

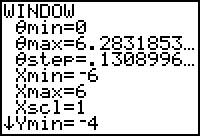
• Enter the following window values:

	Xmin = –6	Ymin = –4
	Xmax = 6	Ymax = 4
	Xscl = 1	Yscl = 1

These window values will be abbreviated as [0, 2 , /24] x [-6, 6, 1] x [-4, 4, 1]. The values will range from 0 to 2 in steps of /24 and the display window will be determined by the x- and y-values.

• Display the graph.

This graph is called a four-leafed rose.

27.2.1 Predict the shape of the graph of r = 3sin(3 ) then verify your prediction by displaying the graph on your TI-83. Click here for the answer.

Finding the Number of Leaves

There is a relationship between the value of n in the polar function r = 3sin(n ) and the number of leaves.

27.2.2 Determine how n relates to the number of leaves in the graph of r = 3sin(n ) by graphing the following polar functions. You will need to adjust to 2 for the functions where n is even and to when n is odd. You may also want to make smaller for the last few functions in order to make the graphs smooth.

Click here for the answer.

27.2.3 How many leaves would you expect in the graph of ? Let and graph the function to verify your prediction. Click here for the answer.

Graphing a Cardioid

• Define the window [0, 2 , /24] x [-6, 6, 1] x [-4, 4, 1].
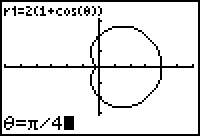
• Graph the polar function r = 2(1 + cos ).

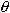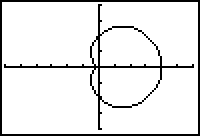
The graph is called a cardioid because it is heart shaped. It is one of several interesting shapes that are common polar functions.

Finding the Slope of a Tangent Line

The Derivative feature in the CALC menu, dy/dx, can be used to find the slope of the line tangent to the cardioid

at .

• Open the CALC menu by pressing [CALC].
• Highlight 2:dy/dx.

• Select this feature by pressing .
• Enter /4. This value will be entered for .

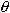• Press to find the derivative dy/dx.

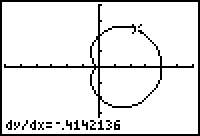
The slope of the tangent line to the cardioid at is approximately -0.414.

Even though the function is in polar coordinates (r, ), the slope is given by , not .

Cardioids and Limaçons

The equation r = a + bcos represents several families of polar curves depending on the relative values of a and b.

27.2.4 Experiment with this equation using a = b, a > b and a < b. How do the relative values of a and b affect the graph? Click here for the answer.