Module 15 - Riemann Sums and the Definite Integral

Module 15 - Answers

Lesson 1

Answer 1

15.1.1

Rectangle x-coordinate y-coordinate Width Height Area

1 1/4 1/16 1/4 1/16 1/64

2 1/2 1/4 1/4 1/4 1/16

3 3/4 9/16 1/4 9/16 9/64

4 1 1 1/4 1 1/4

Total 15/32

Answer 2

15.1.2

Answer 3

15.1.3

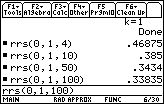
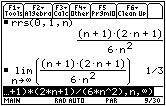
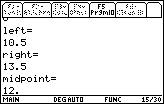
We can read that

Lesson 2

Answer 1

15.2.1

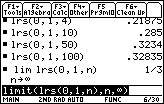
The area under f(x) = x² and above the x-axis between x = 0 and x = 1 is square unit.

Answer 2

15.2.2 The function f(x) = 2x + 1 should be entered into y1 before the program is executed and the Viewing Window should be adjusted to include the interval [0, 3]. The graph below is shown in the window [0, 3] x [0, 10].

The graph illustrating the midpoint rectangles is at left below and the approximate areas found using different types of rectangles is shown at right.

Lesson 3

Answer 1

15.3.1

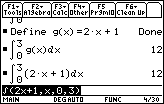
In the screen shown, the value of was found by two methods using the definite integral key. In the first, the function g was defined and then the definite integral was evaluated with its name. In the second, the expression that defines the function was entered directly. In both cases, the area under the graph of g(x) = 2x + 1 and above the x-axis between x = 0 and x = 3 is 12 square units.

Answer 2

15.3.2

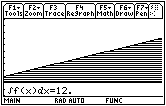
The area under the curve g(x) = 2x + 1 between x = 0 and x = 3 is 12.

Self Test

Answer 1

0.3025

Answer 2

0.2025

Answer 3

Answer 4

Answer 5

Answer 6